1. INTRODUCTION
1.1. A New Theoretical Framework for Insurance Profitability Measurement
The purpose of this paper is to present a new theoretical framework for analyzing the prospective profitability of a property and casualty insurance venture to potential investors. The venture is first modeled as a single policy with underwriting ratios, loss volatility, and payment patterns typical of the business under review. The new paradigm then uses this single policy prototype to generate a multi-year sequence of results. Under this paradigm, a hypothetical company, the Multi-year Company (MYCo.), is established to write the same policy year after year. Its initial capital is supplied by investors. Each year the company writes the same business with the same premium volume, but it has loss results that vary up or down based on random draws from a fixed loss distribution.
After results come in for any year, the company faces several possible scenarios. If the year is profitable, MYCo could retain some of the profit and thereby boost its surplus to a more adequate level. It could also use the profit to pay out some shareholder dividends. If it is flush with retained profits after a series of favorable years, it could distribute any excess surplus as a capital distribution to the investors. In the methodology presented in this paper, those are the only options for applying profits. The approach deliberately prevents profit from being used to write more business or to start new ventures.
If results are unfavorable, the company can simply absorb the loss up to a point, leading to a less adequate level of surplus. However, beyond a certain point, the losses will eat into the surplus to the degree the company will not have enough surplus to continue operations. In that case, MYCo will be liquidated and shareholders will receive whatever surplus is left. If results are deep in the red, the company will be bankrupt with no funds to distribute and claimants may not receive what is contractually owed to them. Note shareholders do not need to contribute any additional funds to make good the shortage of funds in the case of bankruptcy.
The model presented in this paper has a capital management facility that allows the analyst to set shareholder dividends as a percentage of profit. It also features parameters that allow the analyst to define a floor and a ceiling for surplus. Any given sequence can end in either liquidation or bankruptcy. The "ending-badly-but-not-too-badly’ sequences that lead to liquidation are called “liquidation only” (LQO) sequences in this paper. Sequences ending in bankruptcy are called “Bankruptcy” sequences.
The initial capital infusion, the shareholder dividends, and the capital distributions are flows of capital to and from the theoretical shareholders. These payments are called equity flows. (See Robbin 2007). The long-term profitability of MYCo to its investors can then be measured as the internal rate of return (IRR) on these equity flows. To summarize, long-term profitability of an insurance venture can be measured as the return to shareholders who put up capital and stay invested in a company writing the business until it is liquidated or goes bankrupt.
This approach to insurance profitability measurement is called the Robbin-Malhotra (R-M) framework. The defining features of the R-M perspective are listed in Table 1.
A demonstration of this approach will be presented in this paper using a simple multinomial loss model. It is assumed all premiums are paid up-front and all losses are paid after one year. Investment income is earned at a selected risk-free rate on a base of surplus plus premiums. There are no expenses or taxes. The analyst should incorporate appropriate expense, tax, and investment assumptions in any specific application of this framework.
The capital management assumptions that designate a floor and a ceiling on surplus are essential in reflecting real-world constraints. When the surplus for a company becomes inadequate, it will draw regulatory scrutiny and unflattering grades from rating agencies. It will find it hard to write business or it might be legally prevented from doing so. The ceiling of the range reflects the reality that a company with too much surplus will fall under pressure from its stockholders to distribute the excess or demand that it be employed more profitably. Some examples in the paper use wider ranges than are usually seen in practice to highlight their impact on profitability.
One important statistic in R-M analysis is the duration of a sequence, the number of years of writings in the sequence before the business is liquidated or bankruptcy ensues. Different capital management strategies can change the duration of a sequence. So can reinsurance. With R-M analysis, reinsurance can sometimes improve return even if it effectively cedes some profit to the reinsurer. As will be seen, this can happen if the reinsurance increases the average duration of sequences on business that runs at an average net profit.
Several authors (Merton and Perold 1993) emphasize the necessity of incorporating the value of the insolvency put option in the analysis of insurance company capital adequacy and profitability to investors. This put option is associated with bankruptcy scenarios. In those scenarios, the insurance company and its shareholders escape paying the full contractual obligation to claimants. Thus, the average annual loss actually paid is less than the loss expectation from the underlying loss distribution assumed in the model.
The R-M model does reflect the benefit of the insolvency put option to investors, but it also reveals a potentially offsetting adverse bias effect. This effect arises from LQO scenarios. In those scenarios, the final year of the company is punctuated by a large loss that is paid in full. An important observation in R-M analysis is that the duration of an LQO sequence can impact the average annual loss paid by the company over the years comprising that sequence.
Long duration sequences are typically ones in which there are long strings of mostly profitable years with at worst minor reversals. The average loss for long duration sequences is often below the mean. On the other hand, short duration LQO sequences tend to have an average paid loss above the underlying mean. Before any money is made, the sequence ends with a bad loss that is paid in full. The tug-of-war between the impacts of short duration LQO sequences, bankruptcy sequences, and long LQO sequences could end up on either side. Depending on the parameters, the average loss paid out by MYCo could be higher or lower than the theoretical mean of the loss distribution. The selection of capital management parameters can impact the duration of sequences and thus impact the return to shareholders in the R-M framework.
Within this paradigm, it is possible for fairly priced reinsurance to have a beneficial impact on shareholder return. This could happen if the reinsurance provides enough protection to keep the company out of some fraction of the short LQO scenarios. This understanding of the potential benefit of reinsurance is a new insight arising from the R-M perspective.
1.2. Existing Literature
Though there are single policy models which are used to construct multi-year growth models in the literature (Robbin 2007), the authors’ search did not find any model of return on a sequence of random results from a multi-year company. The models of IRR on Equity Flows on a single policy look at results when losses, payout patterns, expenses, and investments are as expected and surplus is set and maintained at a given level of adequacy. These do not evaluate the distribution of returns on a sequence of simulated results. That school of modeling also includes growth models in which the company writes new business each year at a volume that grows at a constant rate. These models do compute calendar year ROE for a growing company, but one with results for each policy that always follow expectations. In such models, there is a growth phase until the model reaches equilibrium when all balance sheet and income statement accounts grow at the fixed growth rate. There is no duration concept: the business grows indefinitely.
There is some work on growth and multi-year modeling in Dynamic Financial Analysis (DFA) (J. Burkett et al. 2010; J. C. Burkett, McIntyre, and Sonlin 2001; D’Arcy et al. 1998; Wiesner and Emma 2000). These models examine premium growth scenarios, investment strategies, and reinsurance programs, and simulate possible results reflecting volatility and correlations between various assets and loss reserve liabilities. Random scenarios are generated, and surplus adequacy is analyzed over a fixed time horizon such as 5 years. These models produce results such as the probability company surplus would fall to the point the company would run afoul of RBC guardrails. The DFA analysis reviewed in J. Burkett et al. (2010) does look at the impact of reinsurance on capital adequacy. D’Arcy et al (1998) and Wiesner and Emma (2000) focused on validating a firm’s operational strategy by reviewing distributions of key variables such as statutory surplus, premium to surplus ratio, and net income over a 5-year time horizon. However, none of the DFA work reviewed considered the return to investors over a multi-year period. Previous authors reviewed the distribution of single-year returns over a string of several years, which is not quite the same thing. Additionally, the DFA literature reviewed by the authors had no significant treatment of excess surplus distributions or the payment of profit-sharing dividends.
Though several papers, books, and study notes discuss advantages of reinsurance, a literature search found none that discussed or proposed a methodology to quantify the potential for reinsurance to improve the return to stockholders over a multi-year period.
The modeling set-up in this paper is also reminiscent of corporate planning models in which several years of expected results are generated. Some of these include payments of shareholder dividends. The authors have also seen models in corporate capital management and investment units looking at the potential benefit to shareholders from buying back stock or raising capital with special notes and debt instruments. However, the authors have not seen these run as multi-year simulations with returns computed for each sequence and the average from a set of such simulated returns used to measure profitability.
The idea of looking at return as an average of returns on long sequences of repeated writings is conceptually related to the school of profitability analysis associated with the information analyst, Kelly. The Kelly (1956) paradigm features a series of bets with the same odds. The gambler is able to vary the amount of the bet as a percentage of their stack of chips. The Kelly analysis focuses on finding the percentage of the bettor’s chips that should be bet each time so as to maximize the expected long-term growth of the stack. The pure Kelly model does not have interim payments back to the gambler’s backer: all winnings stay on the table. There is no explicit backer in the first place. There is also no liquidation short of bankruptcy. The work in this paper has features not found in Kelly. A literature search has not found any insurance model adaptation of the Kelly approach.
The framework of multi-year sequences in this paper can also be fairly viewed as an implementation of Markov chain methodology. In insurance, the primary use of Markov chain approaches has been in the area of reserve risk analysis (Meyers 2019). A literature search turned up no implementation of Markov chain approaches in analyzing shareholder return as is done in this paper.
Though the method in this paper draws on other approaches, none of the previous work in the literature captures and coherently applies the critical elements listed in Table 1, nor have they been applied to studying the impact of capital management and reinsurance on long-term return.
1.3. Organization of the Paper
Chapter 2 will describe the model and include equations and assumptions. Chapter 3 will be a discussion of the impact of reinsurance and include presentation of the IRR distribution results starting with results without reinsurance and then comparing them with results assuming reinsurance had been purchased. The chapter will also include sensitivity analysis showing the impact of changing the reinsurance attachment and limit, and also the percent of the layer placed and the impact of changing the capacity load for the reinsurance. Chapter 4 will be a short summary of the R-M framework and the new insights about the impact of capital management and reinsurance on profitability.
2. A Model of Long-Term insurance Profitability
In this chapter, a simple multi-year model of an insurance company will be constructed and the long-term return to investors will be computed. This company is the Multi-Year Company (MYCo). MYCo writes the same direct premium each year. The premium is the sum of the expectation of the underlying loss random variable and a risk load proportional to the standard deviation of the loss random variable. There are no expenses. Premiums are assumed to be written at the start of each year. Direct losses each year are simulated from a simple discrete distribution. The losses are assumed to be paid out at the end of one year.
Let X denote the direct loss random variable. The direct premium is given as:
In this paper, a very simple example is presented in which the loss distribution is multinomial. The formulas for the mean and standard deviation are:
The specific example in this paper is based on a three-point multinomial. Table 3A details the loss distribution and shows its mean, standard deviation and coefficient of variation. Table 3B documents the derivation of the direct premium as the sum of the underlying expectation plus a risk load proportional to the standard deviation.
The underwriting gain random variable can now be defined. Recall there are no expenses in this demonstration model. Let PREM be the premium computed using Equation 1 as shown in Table 3. Let X(t) be the direct loss simulated in year t. The Underwriting Gain, U(t), in year t is given as:
Next the model is extended to include surplus and investment income. Let SBOP(t) be the surplus at the beginning of the tth time period and SEOP(t) be the Surplus at the end of the period. Invested assets are the sum of the premium plus the beginning-of-period surplus. Assuming a fixed risk-free investment rate of return, r, the investment income INV(t), in the tth period is given as:
Define INC(t) to be the income for the tth time period. In this simple model without taxes, income is the sum of underwriting income plus investment income.
In real-world situations, the analyst should include expenses, taxes, and more detailed treatment of investment income.
The user of the model sets capital management parameters. These include the initial starting surplus, S0, for the company and also a floor and a ceiling for MYCo surplus. In the model the initial surplus is a selected percentage of the standard deviation of direct loss, and the floor and ceiling are selected percentages of the initial surplus.
Let DIV(t) be shareholder dividends paid at the end of the tth period and let Q(t) stand for a distribution of capital at the end of the tth period. The shareholder dividends are assumed to be a percentage of income when income is positive and the beginning surplus is above the initial value. There are no negative shareholder dividends.
The specific values of the capital management parameters and investment rate of return used in the example in this paper are given in Table 4.
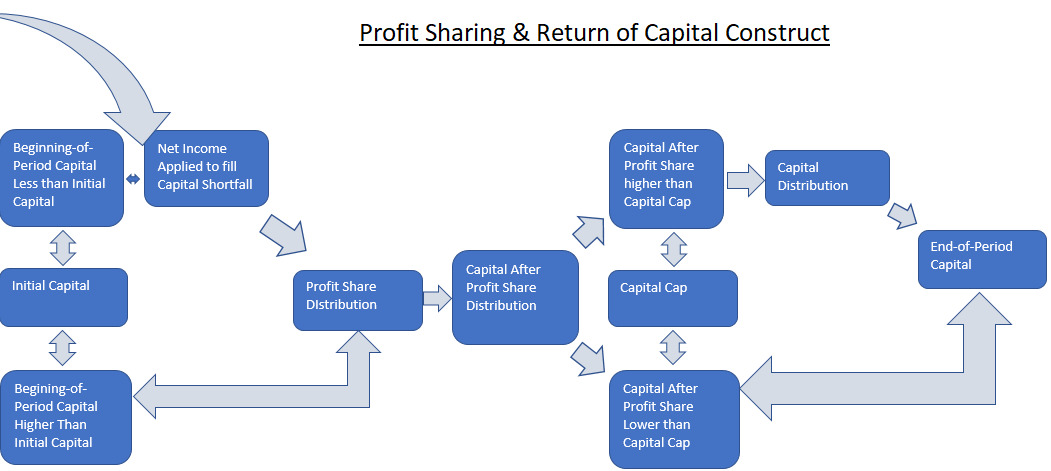
A distribution of capital in this model occurs in two disparate situations. First, when there is excess surplus, that excess is paid out as a capital distribution. The order of calculation is that the shareholder profit-sharing dividend is paid and then a capital distribution is paid if needed. The second situation in which capital is distributed is when the company suffers a loss that would drop the end-of-period surplus below the surplus floor. In that event, the company distributes the remaining surplus to the shareholders so that the end-of-period surplus is zero after the capital liquidation.
If the company suffers such a severe loss that the beginning-of-period surplus plus income for the period sums to a negative number, then the company is bankrupt. There is no capital distribution and the surplus goes to zero. Note there is no recoupment of the shortfall from the investors.
To summarize the equations for capital distributions and the relation between beginning and end-of period surplus,
Equity flows to investors are computed for each year in a multi-year sequence. A positive equity flow is money going to the shareholder. The venture starts off with a negative equity flow equal in magnitude to the initial surplus. The remaining equity flows are equal to the profit-sharing dividends and capital distributions. The sequence in theory is run until the company goes broke or is liquidated.
The Internal Rate of Return (IRR) on the equity flows is computed (see Robbin 2007). Because the structure is set up so that the initial flow is negative and all the rest of the flows are non-negative, the IRR is uniquely determined.
The IRR is computed for several hundred simulated sequences. This gives a distribution of returns to investors.
The next step is to add reinsurance to the model. To prevent confusion, variables may be subscripted with a “DIR”, “CEDED”, or “NET” label as necessary. Net premiums, losses and underwriting gain are equal to direct less ceded:
It is important to note that the net premium computed by differencing as shown in Equation 9 is not the same as applying the premium formula in Equation 1 to net loss. This happens because the standard deviation risk load is not additive. This is not peculiar to standard deviation. Many risk load formulas are not additive. In contrast, the net premium is, by definition, the difference between direct and ceded. The authors do not advocate one risk load or the other. Rather the purpose is to make the reader aware that net premium cannot be expressed via a risk load formula applied to net loss if the risk load is not additive.
Except with respect to surplus, the equations for invested assets, investment income, income, and shareholder dividends apply (Equations 3 through 8) after substituting net premium and net loss in place of direct. As regards the initial surplus, it was set as a percentage of the direct loss standard deviation. One could keep that absolute amount of surplus after the placement of reinsurance, or one could set the initial surplus in proportion to the net loss standard deviation. Later in the section on reinsurance, some analysis of both options will be presented. For now, the reader should regard the initial surplus as a given quantity, not necessarily pegged against net or direct loss. There is no direct surplus and net surplus in this model: only surplus.
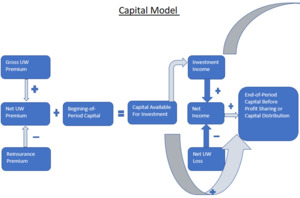
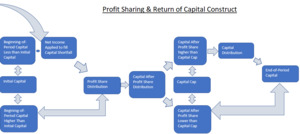
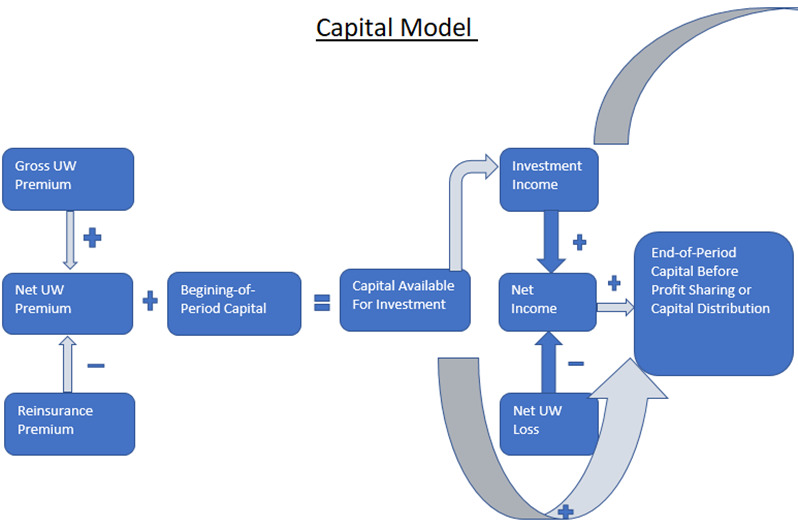
At this point, all the fundamental modeling equations have been given. It is helpful to step back and look at the schematics in Figure 1 and Figure 2 to see how they all relate together. Figure 1 shows how underwriting income and investment income impact surplus. It has an arrow going off the page that denotes capital going to shareholders. Figure 2 shows the profit-sharing dividends and capital distribution relations.
The next step is to show how the net construction works with Stop Loss reinsurance. This is Excess of Loss reinsurance that covers a share of loss excess of an attachment, K, up to a limit, ∆K. The 100% treaty layer is referred to as ∆K excess of K and is written as ∆K XS K. The symbol, ρ, denotes the share covered. In some cases, the notation, ∆K XS K, may be used as a subscript to refer to the treaty layer on a 100% basis. The expectations for treaty layer loss, ceded and net loss are given as:
The attachment in the examples in this paper are a percentage of direct premium and the XOL limits are a percentage of the underlying expectation of direct loss.
The reinsurance on a 100% basis is priced as the sum of the expected layer loss plus a capacity charge which is given as a selected percentage, η, applied to the portion of the layer excess of the expectation. The experience of the authors is that the capacity charge approach is often used in computing an initial estimate of the risk load when pricing an XOL. It at least guarantees pricing indications will not violate Reinsurance Pricing Maxim 1: No Free Cover. In this paper the simple model has no expenses or taxes. To summarize, the formulas for layer premium and the ceded premium are given as:
Values for parameters and the resulting attachment and limit are shown in Table 5. Table 5 also documents the share.
One can now compute the underlying direct, ceded, and net loss expectations and loss standard deviations. These are shown in Table 6.
With this and the coverage parameters one can now derive the Stop Loss Premium as shown in Table 7.
One can now tabulate or compute the Direct, Ceded, and Net Premiums, Expected Losses, and Expected Loss Ratios (ELR). These are shown in Table 8.
Table 8 also shows the underwriting (UW) profit provisions. The profit provisions are effectively the risk loadings for the direct and ceded premiums. However, the profit provision for the net is simply the difference between net loss and net premium, both obtained by subtracting ceded from direct.
One must exercise some care in talking about expectations and profit provisions when in the R-M framework. In Table 8, the expected loss ratio (ELR) is the ratio of expected loss over premium, where the expected loss is the underlying loss expectation obtained from the multinomial.
However, in any given sequence of results for MYCo, the average loss ratio, ALR, defined as the ratio of the average loss over the average premium, will in general not be equal to the ELR. Further the average paid loss ratio, APLR, defined as the average paid loss over the average premium will be different from the ALR in bankruptcy scenarios, because in the year of bankruptcy the company will not pay out the full loss obligation. This is another way to account for the insolvency put. What is important to observe is that capital management parameters may change the duration of a sequence and thus impact its ALR. Knowing the random sequence of simulated losses does not uniquely determine the ALR.
The next step is to generate sequences of results by year. Tables 9-11 show results by year for ten years under three different scenarios. Table 9 shows a scenario (the Survives Scenario) where the insurer survives ten years. Table 10 shows a Bankruptcy Scenario where the insurer experiences bankruptcy on account of adverse loss experience in year 4. Table 11 shows an LQO Scenario where the insurer is liquidated without bankruptcy after the end of year 4. Each of these Tables has two parts. Table A shows the premiums, losses, and underwriting gain. Note there are separate columns for Net Loss and Net Loss Paid. Table B shows the surplus, income, shareholder dividends, capital distributions, and equity flows.
Next comes the Bankruptcy Scenario.
Finally, there is the LQO Scenario:
These demonstration exhibits show ten years, but the last years in the Bankruptcy and LQO scenarios show blanks to drive home the point that the sequence has ended. In more complete runs, many MYCo sequences will go far longer than ten years. The authors used a stopping point of 100 years in subsequent results shown. The IRR in trials of one-hundred-year sequences is effectively the definition of long-term return. It has been found that adding a number of years after that does not have much impact on the return measured. The reason is that discounting over a hundred years with any real interest rate above 1% effectively reduces any tail flows to insignificance. The authors believe that only some low IRR scenarios are impacted by the cut-off.
Another point to observe is that the IRR can be greater than -100% even when a run ends in bankruptcy. This is due to the interim profit dividends and capital distributions paid to the shareholders. In contrast under the Kelly paradigm, if 100% of the stack is wagered repeatedly and there is one bad result, the entire stack is lost, and the gambler’s return is -100%.
The next step is to run the model to generate a large number of random sequences so that one can arrive at an approximate empirical distribution of investor returns. With the distribution of returns one can compute the mean and standard deviation of the shareholder return. In the results that follow, 250 sequences of 100 years were used to generate the distribution of returns. The averages shown are taken over the 250 trials. For returns this makes perfect sense as each sequence generates one multi-year return. Similarly, the average duration is an average of 250 distinct duration values, one for each sequence. However, there is some ambiguity with respect to the average loss ratio as the different sequences have different durations and hence different premium volumes. In what follows, the 250 count numerical averages are used unless otherwise stated.
Table 12 shows the specific parameters used to generate the results in Table 13.
The following table, Table 13, shows the sample averages and sample standard deviations for net underwriting gain, the duration, and the IRR to MYCo Investors by Ceding Percentage.
The example was deliberately chosen with a low treaty attachment of 100% of direct premium and a limit covering the maximum direct loss. This effectively insulates MYCo from any large net losses if the treaty is fully placed. As the large standard deviations for Net UW Gain and IRR at the lower ceding percentage indicate, though very large returns can be enjoyed in some sequences if no reinsurance is placed, the average return is clearly improved by placing 100% of the treaty. Not coincidentally, average duration also rises dramatically in going from 0% reinsurance to a fully reinsured program. Recall the model was run for a sequence of 100 years. So, the resulting 97.9 years average in the 100% placed row means nearly all the sequences went on for the full 100 years. It is also as anticipated that the average net underwriting income is larger for the fully reinsured scenario, even though the strategy without reinsurance yields more profitable results for some set of sequences.
It is helpful to see these relations in graphic form. The following is a graph of Average IRR and Empirical Standard Deviation as a function of Percent Ceded.
The choppy line is to be expected when simulating with a three-point multinomial. Some of the choppiness is also due to the sharp line liquidation boundary. A loss that causes surplus to fall below the floor at one percentage ceded may not lead to liquidation if the percentage ceded is slightly larger. The slightly large cession reduces the net loss just enough to keep the company’s surplus above the floor. The company survives and may go on to achieve a much higher return. The graph also highlights that a win-win effect is possible: a higher average return and a lower empirical standard deviation can be achieved as the ceded percentage rises.
In the next section, the value of reinsurance will be explored under traditional approaches and then from the R-M perspective.
3. The Impact of Reinsurance
The goal in this chapter is to analyze how the purchase of reinsurance by the insurance company could impact the return achieved by insurance company investors. This analysis assumes the reinsurance is priced as the sum of the expected underlying ceded loss plus risk load. The conclusions of this analysis could depend on the magnitude of the risk load. In what follows, the analysis will be done only for an XOL treaty.
The analysis will proceed in stages, looking at different perspectives on the problem. First the analysis will be done using a single-year model with expected values plugged in to yield expected profit and expected return. The next stage will incorporate the insolvency put option. Finally, the R-M framework will be applied to the problem.
The initial thought is that the purchase of reinsurance is at best a necessary evil. It is needed to appease regulators, rating agencies, and prospective consumers. Simplistically viewed, each risk load dollar diverted to reinsurers is a reduction in the provision for net profit. Thus, reinsurance reduces the expected net profit that might then be paid to investors in the form of stockholder dividends or capital distributions.
Now suppose the surplus is unchanged and a quick back of the envelope calculation of return is done with return approximated as the ratio of the profit provision over the surplus. With less profit, the numerator is smaller and the denominator is unchanged. So, the first observation is that the purchase of reinsurance reduces the expected return to shareholders, all else being equal. This is simply due to the reduced leverage when surplus remains fixed and profit is reduced.
What if the surplus is reduced in proportion to the reduction in premium? For a pure quota share with the net and direct profit provisions the same as a percent of premium, the net return and the direct return would be the same, but on a smaller volume of capital. However, one arrives at a different conclusion for an XOL treaty. Assume, as is almost always the case, that the treaty covers a layer of loss that is relatively more risky than the overall direct loss. Indeed, it would be hard to show risk transfer if that were not the case. Thus, it is reasonable to assume the ceded premium for the XOL treaty would have a larger profit provision percentage than the profit provision percentage for direct premium. By subtraction, the net profit would be a smaller percent of net premium than the direct profit provision. To summarize, under the reasonable assumption that the XOL layer loss is relatively more risky than the direct loss, then one should see a net profit provision smaller than the direct profit provision.
If the company reduces capital as a consequence of its XOL reinsurance purchase so the premium-to-surplus ratio stays the same, the expected return declines. The volatility of the return also declines, but whether the overall result is better for the investors depends on their risk-return preferences.
Another option is to reduce the surplus so that the investors achieve the same expected return. Then the question is whether the volatility in return is reduced proportionately. That depends on how volatility is measured. If standard deviation of return is used to measure volatility, then reducing surplus to keep the same expected return leads to a higher volatility of return. In other words, when investors are given the same expected return after reinsurance, the standard deviation of their return is higher than it was for the original direct deal. This is due mathematically to the correlation between net and ceded losses. One could debate the metrics and adjust surplus in different ways, but the conclusion from single-year expected value analysis is that the purchase of reinsurance does not lead to any obvious improvement in the risk-return profile of the shareholder’s investment in the company.
This is demonstrated by example in Table 14. Note there is no underlying loss distribution provided for this example: only the underlying mean and standard deviation are given.
In this example, the company sets the direct risk load (expected profit) at 20% of the standard deviation of direct loss, while the reinsurer sets its risk load at 20% of the standard deviation of ceded loss. The reinsurer in this hypothetical case takes on ceded losses with a higher coefficient of variation (CV) than that for the direct losses. Assuming there is a 75% correlation of ceded and net loss, the CV of net loss is .30, down from a CV of direct loss of .40, a 25% reduction. However, the net profit went down 50% from 8.00 to 4.00. Similarly, under the static single-year model the expected return drops 50%, from 10% to 5%, assuming Capital is unchanged. If capital is reduced to keep the expected return the same at 10%, the standard deviation of return rises from 50.0% to 56.9% due to the reinsurance. These results are sensitive to the correlation between ceded and net Loss. A high correlation was chosen in this example. There should usually be some large correlation between ceded and net loss. On a quota share, the correlation could be 100%. With an XOL Treaty, small direct losses might not lead to any ceded loss and the resulting net loss would also be small. Only when direct losses are big would reinsurance kick in. So small ceded losses are associated with small net losses, and also large ceded losses are associated with the large net losses. Thus, even with an XOL Treaty, there should be a significant correlation between ceded and net loss. The example demonstrates that in the static single-year framework, the purchase of reinsurance that removes risk and is fairly priced does not lead to any clear advantage for the investors.
It gets worse. The value of reinsurance to investors looks even more dubious if the effect it has on the implicit insolvency put option is considered. The insolvency put option has been an important aspect of insurance capital valuation from the investor perspective going back to Merton and Perold (1993). The observation is that insurance company investors can only lose the capital they have already put in. If losses are so severe as to totally deplete the corporate capital, the investors are shielded from any additional obligations. Thus, the investors do not have to cover the full downside potential of the contracts issued by the company. From this perspective, a poorly capitalized insurance company is good for its investors. Another way to see this is to observe that “poorly capitalized” is another way of saying “highly leveraged”. The asymmetry where investors reap the profits when outcomes are favorable but don’t lose the full amount when outcomes are adverse leads to a reduction in the expected loss that will be covered by the investors. This is one intuitive way of quantifying the value of the insolvency put option.
Reinsurance seems even less useful to investors when the insolvency put option is considered. Again, continuing the analysis in a single year static model framework, one sees that reinsurance will act to reduce the frequency and/or severity of net loss. It will cover some scenarios so that the full loss obligation is covered even if the company would have gone broke in a direct scenario without reinsurance.
Consider the example in Table 15. The probability of bankruptcy is approximated as the probability of being above the sum of capital plus premium. (A more refined treatment would include an adjustment for a year of investment income). That probability in this example computed using the normal distribution. Conditional severity is assumed to be one standard deviation. Comparing the “Direct” and “Net – No change in Capital columns”, one can see that reinsurance is effective at reducing the frequency and severity of bankruptcy of the insurance company. However, it also acts to reduce the value of the insolvency put option and thus would tend to reduce return to investors.
Note when capital is not reduced, the value of the insolvency put option gets rounded to zero in this example. This happens because the odds of bankruptcy drop to near zero as a consequence of having such a relatively large amount of capital covering loss that has been reduced by protective reinsurance. Cutting capital in half in this example raises the expected return. It also recovers more than the initial value of the insolvency put option offsetting the reduction due to reinsurance. However, the odds of bankruptcy are much higher than in the direct case. To summarize, if capital is reduced in response to the purchase of reinsurance, one can raise the return but at a cost of increasing volatility. How this all plays out depends on the specifics. But it is not immediate that the combination of placing reinsurance and reducing capital leaves the investors better off from a risk-return perspective.
This changes under the R-M Multi-year model. The analysis gets more complicated once the model has a capital floor that triggers liquidation even when there is still sufficient capital to stave off bankruptcy. Capital floors are of more than a theoretical concern. They correspond to a threshold below which capital would be considered inadequate to write the business. If capital is inadequate, regulators or rating agencies could make it impossible for the company to go on without intervention.
When average annual losses are examined in an LQO scenario of short duration, they tend to be higher than the theoretical mean of the losses on their own. The reverse is true for longer duration sequences. If the sequence ends relatively quickly and on a bad note, the average loss in the sequence tends to be higher than the underlying mean. But, if the sequence goes on for a while without running into an LQO or bankruptcy, the average loss paid out will tend to be fairly low, even below the underlying mean.
For an example of this, consider a sequence of rolls of a fair die that will end when the first 6 is rolled. It can be easily proved the average will be less than 3.5, the underlying mean, if the sequence goes on for more than 6 rolls.
There are offsetting effects revealed in R-M multi-year random sequential analysis. The average net loss paid is above the underlying theoretical mean for short LQO sequences. However, for long LQO sequences the average loss paid tends to be below that mean. A given sequence eventually ends in liquidation or bankruptcy. However, from an IRR on Equity Flows perspective what happens after a hundred years has a negligible impact on the return. Basically, most long-lasting sequences generate a nice return and have average loss payments below the mean.
Reinsurance can transform a Bankruptcy event into an LQO event or save the business to continue into the future. While reinsurance can thus diminish the value of the insolvency put, it can also promote an increase in the relative number of long LQO sequences. That leads to a lower average paid loss and a higher average return. The important conclusion is reached that if capital is managed appropriately and a reinsurance purchase is made that acts to stave off short LQO scenarios, then the average net loss paid in the multi-year sequences (as a ratio to net premium) could be below the underlying single-year expected direct loss ratio. If that happens often enough, then the expected value of average losses paid under the sequences is reduced and average return is increased.
How all these offsets play out depends on the capital management parameters and the reinsurance deal. If a company can reinsure away all adverse outcomes at a reasonable price, it can generate a higher average return to shareholders under this long-term perspective. This can happen even when reinsurance is counter-indicated from the single-year static view. Thus, the R-M framework provides a different way of seeing how reinsurance might potentially benefit shareholder returns. This is a new and important explanation for the value of reinsurance.
The conclusion from the summary results in Table 13 and Graph 1 is that Stop Loss reinsurance can improve long-term profitability under certain conditions. It must provide sufficient protection to prevent quick “ending badly” sequences from happening too often, because those lead to liquidations and no chance of recouping profits in subsequent years. Yet, it cannot be too expensive. From an intuitive point of view, this is hardly surprising. Saying that investors profit from reasonably priced reinsurance that provides adequate protection to keep the company going doesn’t sound controversial. However, the authors know of no other model in the literature that illustrates and allows the analyst to quantify this result. Reinsurance can improve long-term profitability despite its adverse impact on the value of the insolvency put option.
4. Sensitivity Analysis
This section presents sensitivity analysis of the impact of various capital management and reinsurance parameters on long-term return. The capital management parameters are the initial capitalization level, the floor and ceiling for capital, and the percentage selected for profit sharing dividends. The reinsurance parameters are the attachment and limit for a Stop Loss treaty along with the percentage of the layer ceded. The sensitivity results form the basis for qualitative observations about how to optimize long-term return. The interaction between the effects of these parameters suggests effective capital management policy should be made in tandem with reinsurance strategy. The R-M framework can be applied to quantify risk-return characteristics of various capital management and reinsurance strategies with respect to the long-term return.
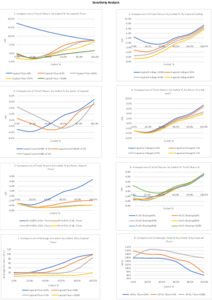
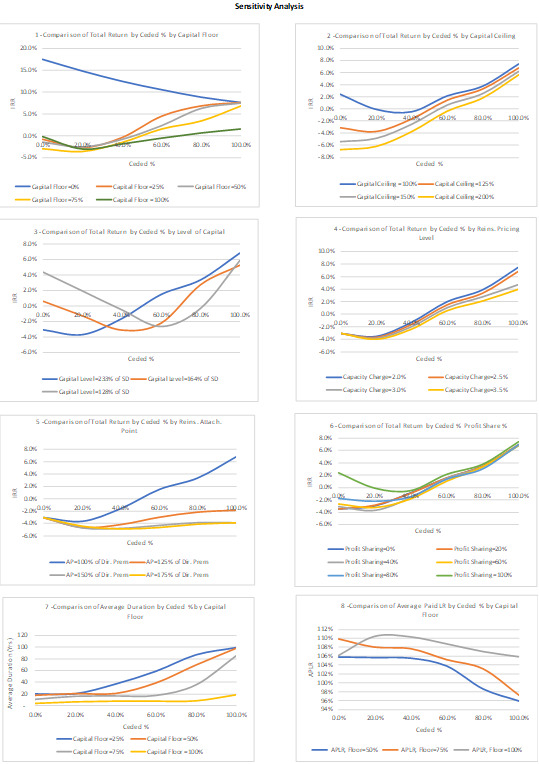
A select set of sensitivity results is shown in a series of charts in Table 16. The “x-axis” in each chart is the percentage of the Agg Stop layer ceded. The “y-axis” in the first six charts is the average IRR. For the seventh chart, the “y-axis” is the average duration and for the eighth it is the average paid loss ratio. Within each chart there are three to six separate lines with different values of the variable subject to sensitivity examination. In that sense the charts are not strictly sensitivity charts, but rather charts that show a comparison of graphs based on different values of one of the variables.
Chart 1 shows a comparison of return at various capital floors (minimum capital) on insurance company capital. Without reinsurance, the “zero” capital floor has the highest average return. Having no capital floor, there are no LQO sequences and the value of the insolvency put is maximized. So, if the company is able to operate when severely undercapitalized, the stockholders can make a very handsome expected return. Adding in reinsurance just lowers the average return. Of course, it is highly unrealistic for the company to operate with extremely inadequate capital. Regulators will seize the company. So, the other lines in the graph corresponding to different capital floors are more realistic. Chart 1 shows returns with no reinsurance are very low or even negative with capital floors of 25%, 50%, 75%, and 100% of initial surplus. As shown in Chart 1 reinsurance at first reduces the return for low percentages ceded. However as larger percentages are ceded, reinsurance eventually leads to improved returns. The curves coalesce except for the 100% floor. This happens because the low-attaching Aggregate Stop Loss cover in our example eventually protects the lower surplus floors from being breached. The management of a company needs to balance how much of a hit it can absorb before liquidation will be forced on it, what it needs to pay to get a low-attaching cover, and the share it needs to place to protect it from hitting a capital liquidation trigger.
Similarly Chart 2 compares total long-term returns at various maximum capital or capital ceiling strategies requiring return of any capital excess of the ceiling back to the investors through a profit sharing and return of capital strategy. As expected, a capital management strategy of retaining less capital leads to higher profitability in the long run for shareholders.
Chart 3 compares the return graphs for different initial levels of capitalization. If the initial surplus is fairly high, the return to shareholders suffers because they get minimal benefit from the insolvency put option. Adding reinsurance initially makes things worse. It sends profit out the door and doesn’t sufficiently extend the duration of LQO sequences. However, past a certain point, when a sufficient percentage is ceded, the return starts to rise for all the curves. Once reinsurance becomes effective at forestalling liquidation from capital inadequacy after a large direct loss, the company survives to generate profits over a longer duration and this improves return to shareholders. This suggests the company management should try to find the sweet spot of raising adequate but not redundant initial capital and place sufficient reinsurance to be on the increasing part of the curve. Of course, the results in the chart depend on the low-attaching aggregate.
Chart 4 compares the total return by reinsurance pricing level. As expected, long-term returns are lower for higher-priced reinsurance. The R-M perspective highlights the problem that low-attaching reinsurance helps improve return, but it cannot be too expensive or it will have a negative effect. None of this is surprising or in contravention of common wisdom.
Chart 5 compares returns at various reinsurance attachment points. It shows that for Stop Loss reinsurance selection of an attachment point is critical in making the reinsurance strategy profitable for MYCo investors. Attachments that are too high do not provide enough protection.
Chart 6 compares shareholder return graphs for different profit-sharing dividend percentages. These graphs show an interesting interaction with reinsurance. Once there is enough reinsurance protection, the return to shareholders improves as they are given a higher percentage of the profits.
Chart 7 compares duration graphs for different capital floors. As anticipated, the duration rises for capital floors or 25%, 50%, and 75% as the percentage of reinsured layer ceded rises. The reinsurance eventually protects the company from liquidation and that raises the return.
Chart 8 shows average paid loss ratio graphs for different capital floors. The loss ratio average is computed as an average of the average loss ratios of the 250 sequences. Since each of the sequences has a different duration, the volume of premium and loss is different for each. This numerical average approach was taken to keep the values consistent with the IRR averages. In any real example, it is also useful to look at the overall premium-weighted average of the loss ratios. The average loss ratios are initially higher for the 75% surplus floor. This is to be expected as it leads to a relatively larger number of short LQO scenarios. However, as the reinsured share increases, the average loss ratio declines as the reinsurance acts to reduce the number of short LQO sequences. For the 100% Surplus Floor scenario we do not see a significant loss ratio decline as reinsurance ceded % increases as reinsurance has less of an impact in reducing LQO sequences when capital requirements are very stringent.
5. Conclusion
The reader has seen how the R-M framework provides a new measure of profitability of an insurance venture based on construction of associated multi-year sequences of results for a multi-year company, MYCo. Underwriting results each year are based on a fixed premium and a loss selection drawn randomly from a fixed distribution characteristic of the venture. The company starts with an initial surplus provided by investors. The surplus changes year to year based on underwriting gains and on a set of pre-selected capital management rules. These govern the payment of shareholder profit-sharing dividends and the distribution of excess surplus. The capital management module also features a surplus floor that triggers liquidation of the company even if it is not completely bankrupt. This produces a sequence described as a Liquidation Only Sequence (LQO) sequence. Return for each sequence is measured as the IRR on the equity flows to the investors. The reader has learned how short LQO sequences impair long-term return. The reader has also seen how reinsurance can be folded into this model and how it can lead to changes in the duration of different sequences. It was demonstrated by example that the return to shareholders could be improved by the purchase of fairly priced reinsurance that provides sufficient protection. This is an intuitive result but one not shown with other measures of return found in the literature. The charts in Table 16 portrayed the need to balance adequate reinsurance protection reinsurance price, reinsurance placement percentage, surplus adequacy, capital floors, shareholder dividend levels, and capital distributions in order to optimize shareholder return. The ability of the R-M framework to incorporate and quantify the impact of capital management and reinsurance in the calculation of return to shareholders is a critical advantage of this approach.
Much research needs to be done. The model needs to be made more realistic with the addition of expense, taxes, and multi-year loss payout patterns. A step beyond that is to incorporate volatility in the loss reserves and to also reflect the impact of loss portfolio transfers. One other path for future investigation is to look at the returns for investors who do not stay invested until liquidation but who stay a certain number of years and then bow out.
Another fruitful area for research would be to extend the single venture multi-year model into a multi-year model of a multi-line company. Such a model might incorporate calendar year effects across lines as well as correlation effects between lines and it would have rules for liquidation of unprofitable lines in addition to liquidation of the overall company.
Overall, it is hoped this new framework will be regarded as a major advance in the analysis of insurance profitability. Ideally it will be a welcome addition to the actuarial toolkit that may help actuaries gain a bigger seat at the table during internal corporate discussions about capital management and reinsurance strategy.
Abbreviations and notations
CV – Coefficient of Variation
DFA – Dynamic Financial Analysis
IRR - Internal Rate of Return
LQO - Liquidation Only
ROE - Return on Equity
Biographies of the Authors
Ira Robbin is an Associate of the Casualty Actuarial Society. He has served in actuarial thought leadership and management roles in several major insurance and reinsurance companies. He has developed new theories, written papers, and delivered presentations on pricing, reserving, return on equity, credibility, loss development, and risk capital. He has a PhD in Math from Rutgers University and a Bachelors degree in Math from Michigan State University.
Atul Malhotra is a Fellow of the Casualty Actuarial Society. He is a Sr. Director & Actuary at Liberty Mutual Insurance Group. He has over 20 years of experience in the P&C industry in the areas of Pricing & Reserving. Mr. Malhotra has coauthored papers on topics in P&C insurance. He holds a Bachelors in Technology from the Indian Institute of Technology and an MBA from the University of New Hampshire.
Disclaimers
Opinions expressed in this paper are solely those of the authors. They may or may not be consistent with the views of current or prior clients or employers of the authors. No warranty is given that any formula or assertion is accurate. No liability is assumed whatsoever for any losses, direct or indirect, that may result from use of the methods described in this paper or reliance on any of the views expressed therein.